The Astrolabe Project

Hartmann’s Practika is a collection of writings by the 16th century instrument maker Georg Hartmann. These detail the steps to design a range of sundials, and extensive notes on astrolabe construction. Hartmann begins by demonstrating the geometrical construction techniques he will be using. Given how complex some of the design work will be, this seems a good place to start

Adapted from Hartmann:

Method 1: Erecting a centered perpendicular.

Given line AB: Place the pivot of the compass at point A and draw two arcs, one above and one below the line as shown. Without changing the size of the compass, move the compass pivot to point B and draw two more arcs, intersecting the first two arcs. With a straight-edge, draw a line through the two intersections. The resulting line will be perpendicular to AB and centered between points A and B.

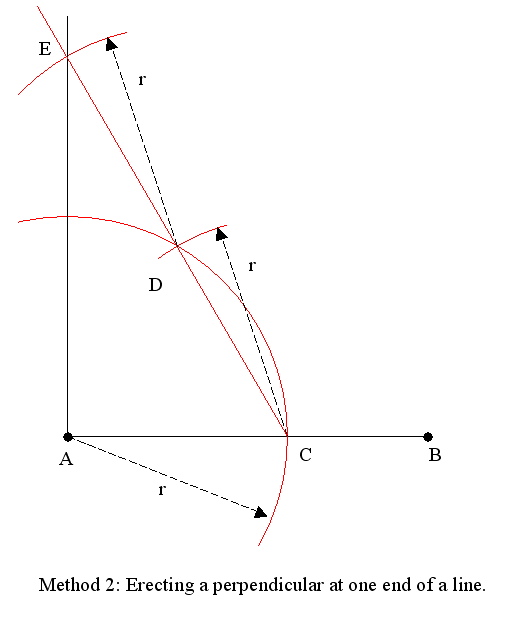
Method 2: Erecting a perpendicular at one end of a line.

Given line AB: Place the pivot of the compass at point A and draw an arc of radius r as shown. Mark the point where the arc crosses AB as point C. Keeping the compass set to radius r, move the pivot to point C and draw another arc, intersecting the first arc. mark this intersection point D. Using a straight edge, draw a line from point C past point D. Keeping the compass set to radius r, move the pivot to point D and draw another arc intersecting line CD at point E. Using a straight edge, draw a line from point A to point E. Line AE is perpendicular to line AB.

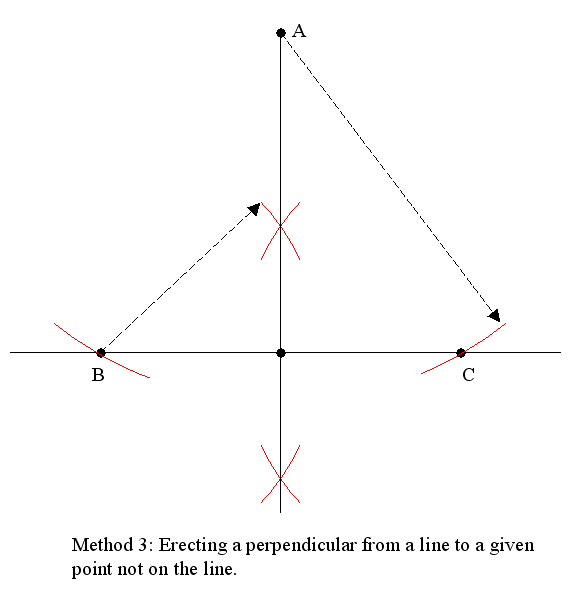
Method 3: Erecting a perpendicular from a line to a given point not on the line.

Given a line and point A not on the line: Place the pivot of the compass at point A and draw an arc intersecting the line in two places. Mark these intersections as point B and point C. Place the pivot of the compass at point B and draw two arcs, one above and one below the line as shown. Without changing the size of the compass, move the compass pivot to point C and draw two more arcs, intersecting the first two arcs. With a straight-edge, draw a line through the two intersections. The resulting line will be perpendicular to line BC and pass through point A.

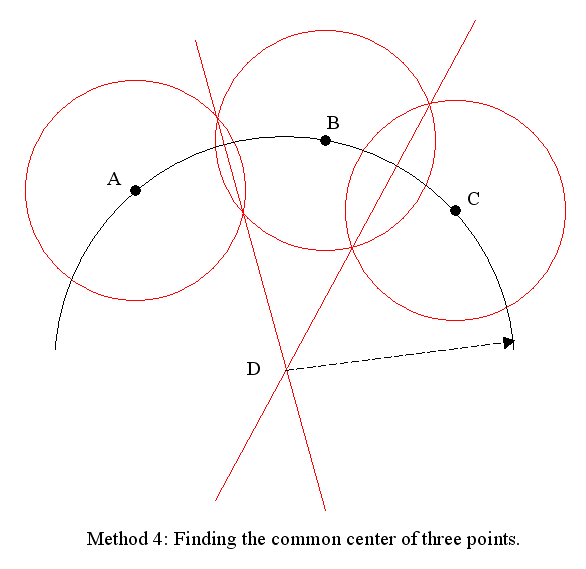
Method 4: Finding the common center of three points.

Given three points A, B, and C, not on a line: Place the pivot of the compass at point A and draw an circle of radius r as shown. Repeat for point B and Point C. Radius r should be set such that the three circles overlap as shown. With a straight-edge, draw lines through the two sets of intersections. These two lines will cross at point D, which is the common center of points A, B, and C.

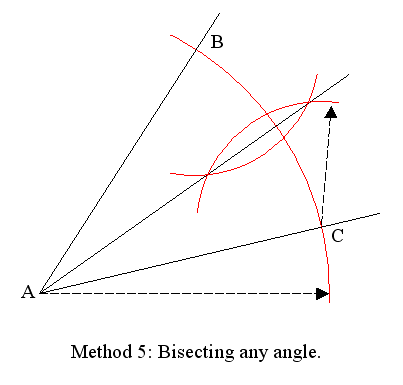
Method 5: Bisecting any angle.

Given angle BAC where point A is the point of the angle: Place the pivot of the compass at point A and draw an arc intersecting the sides of the angle as shown. Move the compass pivot to point B and adjust the compass radius to just past the center of BC. Draw an arc as shown. Keeping the compass set to the same radius, move the pivot to point C and draw a second arc. With a straight-edge, draw a line through the two intersections and point A. This line bisects the angle BAC.

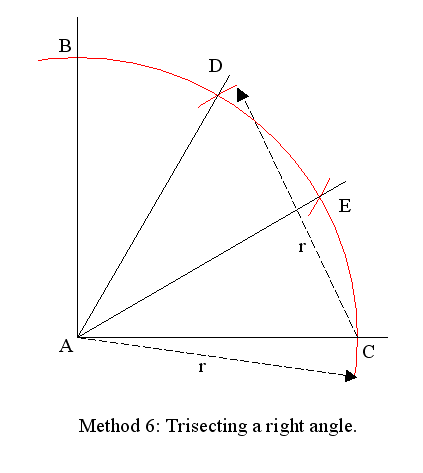
Method 6: Trisecting a right angle.

Given right angle BAC where point A is 90 degrees: Place the pivot of the compass at point A and draw an arc of radius r intersecting the sides of the angle as shown. Without adjusting the compass radius, move the compass pivot to point B and draw an arc that intersects the first arc as shown. Mark this as point E. Move the compass pivot to point C and draw another arc. Mark this intersection as point D. With a straight-edge, draw lines AD and AE. These lines trisect the angle BAC.